Page
maison de Simon Plouffe Home Page
La tête pleine de chiffres / A head full of numbers
au complet ici :
complete book here :
Formulas for the n'th decimal digit of the square root of 2 and 10
gives the decimal of rank k : r3(n) : is the
number of ways of writing a non-negative integer n as a sum of 3 squares : sequence A005875 of the OEIS catalog
A formula for the n'th digit of
and Pi in binary
Time line
1983 :1991 : First version of GFUN
1992
:
1992 : The
n'th Tribonacci number is :
1992 :Master
Thesis : Approximation de fonctions génératrices et quelques
conjectures
1993 : Exact formulas for integer sequences
1995 : ![]()
1995 :
Publication de : The encyclopedia of Integer Sequences
1995
: Ouverture du Inverse Symbolic Calculator
1996 : Algorithm for Pi in base 10
1996 :
Computation of the 10000'th Bernoulli number (with Greg
Fee)
1998 : Ouverture
de l'Inverseur de Plouffe - Plouffe Inverter
1998 : Integer
relations :
2001 :
Fibonacci numbers and plants (and other radio and tv)
2001 :
Computation of the 750000'th Bernoulli number
2006 : New
formulas for Pi and 1/Pi :
2006 : Experiments modulo 1:
2010 :Interesting
approximations
2011 : A formula
for the mass ratio of the neutron/proton :
2011 : 148000
empirical formulas found in the OEIS database
2014 : Strange
formula for some algebraic numbers:
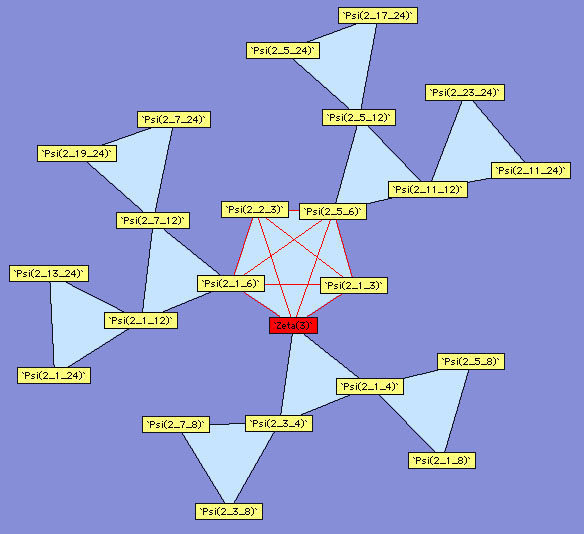
2014 : A formula
for the Zeta function :

2016 : Formula for primes using irrational numbers
2022 : A formula for the n'th digit of Pi (decimal or binary)
2023 : Pi and the primes
2023 : Efficient formulas for Zeta(2n+1)
2023 : OEIS conjectured formulas
Other tables and graphics:
Constants
Binary expansion of 4th degree algebraic numbers
Generalized expansion of real numbers
Integer Relations graphics
Les nombres de Fibonacci et les plantes
Prime numbers in different bases
Lots of graphics
OEIS tables
Other graphics mod 1
Old graphics made by hand
OEIS formulas extended with rational fractions
OEIS with recurrences
OEIS graphs
Numbers reverses in graphics

